I actually don’t have nightmares about maths exams, though they caused me many years of stress and tears in high school. It’s English that keeps coming back to haunt my dreams – scenarios such as having to re-sit the HSC papers otherwise they’ll take my degrees and years of work experience off me because I wasn’t validly in them. Or something.
I got a peek at the Standard and Advanced maths exams while supervising them Monday. They were the old Maths in Practice, Maths in Society and 2 Unit maths subjects from before the turn of the century. I did two unit in year 10, so that content was 26 years ago for me… gosh. I should have dopped maths after the 3 unit paper in year 11, but no, I was convinced (by who IDK) to continue to 4 unit and the horrors that came with that orange text book. As I said to the other supervisors on Monday, I failed the 4 unit exam by marks but it still scaled to mid nineties, so yeah, I’m told it was worth the feelings of failure.
I also confessed to the other supervisors, when they were saying they don’t understand why anyone would try to cheat, that I’d cheated in one maths test once – a Maths Olympiad paper in grade five, the sort of maths competition where the content was part of the HSC syllabus but they threw it at primary school kids to see what they could do. I really don’t know where the pressure came from in primary school for me. I felt like I HAD to top that exam for my school otherwise I wouldn’t be doing what I was supposed to do. Just like how I cried when I didn’t “top” the Basic Skills Test maths (Think NAPLAN now) for my school. Even though it wasn’t a test that was meant to impact anything. This is also the girl who cried in Kindy cos she had to stay home sick because she thought she’d get behind, even though she was reading and comprehending at three years old.
Apparently I was more boisterous before Kindergarten and then my school reports from grade one started calling me timid. And I blame the strict Kinder teacher. While she may never have had to discipline me in her classroom, I saw what happened to other kids – she smacked them and put them in the store room and other things that terrified me – and that was enough to make me submissive and scared.
Isn’t it fun to reflect on what made you the way you are today?
Discover more from phonakins
Subscribe to get the latest posts sent to your email.
Sorry, I had to respond here as finally deactivated that account.
Yes I can show you how – apologies that I can’t use proper symbols here.
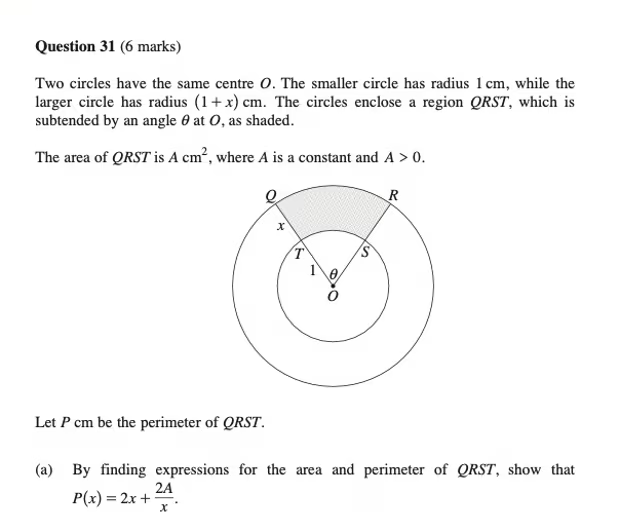
To prove that P(x) = 2x + \frac{2A}{x}, we need to find expressions for the area and perimeter of the region QRST.
Area of QRST
The area of the sector ORS of the larger circle is given by:
Area of ORS = \frac{1}{2} (1+x)^2 \theta
The area of the sector OTQ of the smaller circle is:
Area of OTQ = \frac{1}{2} (1)^2 \theta = \frac{1}{2} \theta
The area of QRST is the difference between these two areas:
A = \frac{1}{2} (1+x)^2 \theta – \frac{1}{2} \theta
Simplifying, we get:
A = \frac{1}{2} ((1+x)^2 – 1) \theta = \frac{1}{2} (x^2 + 2x) \theta.
Perimeter of QRST
The perimeter consists of the arc lengths QR and TS, plus the straight lines QT and RS.
* Arc length QR = (1+x)\theta.
* Arc length TS = 1\cdot\theta = \theta.
Since QT = RS = x, the total perimeter is:
P(x) = (1+x)\theta + x + x + \theta = (1+x)\theta + 2x.
Equating Area to Constant
Given that the area A = A_{\text{constant}} > 0, we have:
A = \frac{1}{2} (x^2 + 2x) \theta.
Solving for \theta, we get:
\theta = \frac{2A}{x^2 + 2x}.
Substituting Back into Perimeter
Substitute \theta = \frac{2A}{x^2 + 2x} into the expression for perimeter:
P(x) = (1+x)\left(\frac{2A}{x^2 + 2x}\right) + 2x.
Simplifying:
P(x) = (1+x)\left(\frac{2A}{x(x+2)}\right) + 2x.
P(x) = 2A\left(\frac{1+x}{x(x+2)}\right) + 2x.
Simplify further:
P(x) = 2A\left(\frac{1}{x}\right) + 2x.
Thus, we confirm that:
P(x) = 2x + \frac{2A}{x}.
I could have done that in year ten but omg my eyes just glaze over now 😩 I’ll get you to tutor the nibbling in a few years